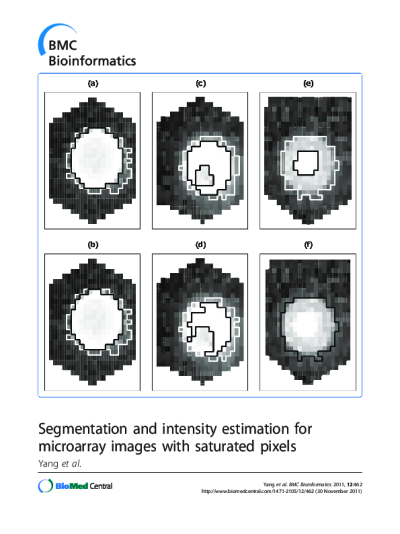
Background: Microarray image analysis processes scanned digital images of hybridized arrays to produce the input spot-level data for downstream analysis, so it can have a potentially large impact on those and subsequent analysis. Signal saturation is an optical effect that occurs when some pixel values for highly expressed genes or peptides exceed the upper detection threshold of the scanner software (2¹⁶ - 1 = 65, 535 for 16-bit images). In practice, spots with a sizable number of saturated pixels are often flagged and discarded. Alternatively, the saturated values are used without adjustments for estimating spot intensities. The resulting expression data tend to be biased downwards and can distort high-level analysis that relies on these data. Hence, it is crucial to effectively correct for signal saturation.

Results: We developed a flexible mixture model-based segmentation and spot intensity estimation procedure that accounts for saturated pixels by incorporating a censored component in the mixture model. As demonstrated with biological data and simulation, our method extends the dynamic range of expression data beyond the saturation threshold and is effective in correcting saturation-induced bias when the lost information is not tremendous. We further illustrate the impact of image processing on downstream classification, showing that the proposed method can increase diagnostic accuracy using data from a lymphoma cancer diagnosis study.

Conclusions: The presented method adjusts for signal saturation at the segmentation stage that identifies a pixel as part of the foreground, background or other. The cluster membership of a pixel can be altered versus treating saturated values as truly observed. Thus, the resulting spot intensity estimates may be more accurate than those obtained from existing methods that correct for saturation based on already segmented data. As a model-based segmentation method, our procedure is able to identify inner holes, fuzzy edges and blank spots that are common in microarray images. The approach is independent of microarray platform and applicable to both single- and dual-channel microarrays.

A least total area of triangle method was proposed by Teissier (1948) for fitting a straight line to data from a pair of variables without treating either variable as the dependent variable while allowing each of the variables to have measurement errors. This method is commonly called Reduced Major Axis (RMA) regression and is often used instead of Ordinary Least Squares (OLS) regression. Results for confidence intervals, hypothesis testing and asymptotic distributions of coefficient estimates in the bivariate case are reviewed. A generalization of RMA to more than two variables for fitting a plane to data is obtained by minimizing the sum of a function of the volumes obtained by drawing, from each data point, lines parallel to each coordinate axis to the fitted plane (Draper and Yang 1997; Goodman and Tofallis 2003). Generalized RMA results for the multivariate case obtained by Draper and Yang (1997) are reviewed and some investigations of multivariate RMA are given. A linear model is proposed that does not specify a dependent variable and allows for errors in the measurement of each variable. Coefficients in the model are estimated by minimization of the function of the volumes previously mentioned. Methods for obtaining coefficient estimates are discussed and simulations are used to investigate the distribution of coefficient estimates. The effects of sample size, sampling error and correlation among variables on the estimates are studied. Bootstrap methods are used to obtain confidence intervals for model coefficients. Residual analysis is considered for assessing model assumptions. Outlier and influential case diagnostics are developed and a forward selection method is proposed for subset selection of model variables. A real data example is provided that uses the methods developed. Topics for further research are discussed.

Value-added models (VAMs) are used by many states to assess contributions of individual teachers and schools to students' academic growth. The generalized persistence VAM, one of the most flexible in the literature, estimates the ``value added'' by individual teachers to their students' current and future test scores by employing a mixed model with a longitudinal database of test scores. There is concern, however, that missing values that are common in the longitudinal student scores can bias value-added assessments, especially when the models serve as a basis for personnel decisions -- such as promoting or dismissing teachers -- as they are being used in some states. Certain types of missing data require that the VAM be modeled jointly with the missingness process in order to obtain unbiased parameter estimates. This dissertation studies two problems. First, the flexibility and multimembership random effects structure of the generalized persistence model lead to computational challenges that have limited the model's availability. To this point, no methods have been developed for scalable maximum likelihood estimation of the model. An EM algorithm to compute maximum likelihood estimates efficiently is developed, making use of the sparse structure of the random effects and error covariance matrices. The algorithm is implemented in the package GPvam in R statistical software. Illustrations of the gains in computational efficiency achieved by the estimation procedure are given. Furthermore, to address the presence of potentially nonignorable missing data, a flexible correlated random effects model is developed that extends the generalized persistence model to jointly model the test scores and the missingness process, allowing the process to depend on both students and teachers. The joint model gives the ability to test the sensitivity of the VAM to the presence of nonignorable missing data. Estimation of the model is challenging due to the non-hierarchical dependence structure and the resulting intractable high-dimensional integrals. Maximum likelihood estimation of the model is performed using an EM algorithm with fully exponential Laplace approximations for the E step. The methods are illustrated with data from university calculus classes and with data from standardized test scores from an urban school district.

It is common in the analysis of data to provide a goodness-of-fit test to assess the performance of a model. In the analysis of contingency tables, goodness-of-fit statistics are frequently employed when modeling social science, educational or psychological data where the interest is often directed at investigating the association among multi-categorical variables. Pearson's chi-squared statistic is well-known in goodness-of-fit testing, but it is sometimes considered to produce an omnibus test as it gives little guidance to the source of poor fit once the null hypothesis is rejected. However, its components can provide powerful directional tests. In this dissertation, orthogonal components are used to develop goodness-of-fit tests for models fit to the counts obtained from the cross-classification of multi-category dependent variables. Ordinal categories are assumed. Orthogonal components defined on marginals are obtained when analyzing multi-dimensional contingency tables through the use of the QR decomposition. A subset of these orthogonal components can be used to construct limited-information tests that allow one to identify the source of lack-of-fit and provide an increase in power compared to Pearson's test. These tests can address the adverse effects presented when data are sparse. The tests rely on the set of first- and second-order marginals jointly, the set of second-order marginals only, and the random forest method, a popular algorithm for modeling large complex data sets. The performance of these tests is compared to the likelihood ratio test as well as to tests based on orthogonal polynomial components. The derived goodness-of-fit tests are evaluated with studies for detecting two- and three-way associations that are not accounted for by a categorical variable factor model with a single latent variable. In addition the tests are used to investigate the case when the model misspecification involves parameter constraints for large and sparse contingency tables. The methodology proposed here is applied to data from the 38th round of the State Survey conducted by the Institute for Public Policy and Michigan State University Social Research (2005) . The results illustrate the use of the proposed techniques in the context of a sparse data set.